MSHU Устройство простых гармонических колебаний

7.1.4.- ВИБРАЦИИ И КОЛЕБАНИЯ 14.1.4.- ВИБРАЦИИ И КОЛЕБАНИЯ
SIMPLE HARMONIC MOTION UNIT - MSHU

ИННОВАЦИОННЫЕ СИСТЕМЫ

This Simple Harmonic Motion Unit, "MSHU", allows many experiments using several pendulums and devices, such as different types of pendulums (simple, bifilar, trifilar and Kater’s pendulum) and springs.

Посмотреть общее описание

Расширения

ICAI

Программное Обеспечение для Интерактивного Компьютерного Обучения
MSAC

Модульная Система Сбора и Управления

НОВОСТИ ПО ТЕМЕ

EDIBON and its commitment to European Skills
EDIBON and its commitment to European Skills
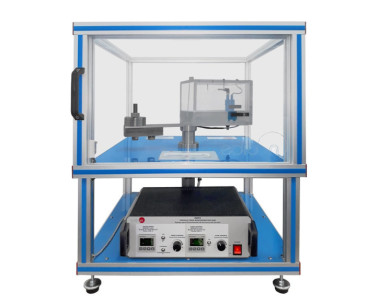
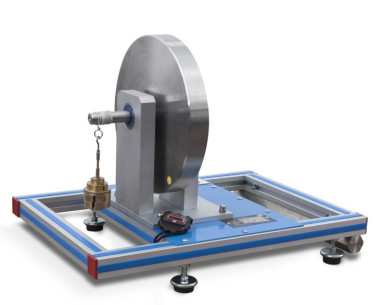
At EDIBON, we are committed to strengthening skills as a strategic pillar for the competitiveness and social well-being of the European Union. Proper training not only boosts the economy but also enables individuals to fully participate in society and democracy.
Читать дальше
Setting up at South Mountain Community College (MARICOPA)
Setting up at South Mountain Community College (MARICOPA)
We have moved to the engineering lab of South Mountain Community College (MARICOPA) to install some of our units. With these new units, we hope that students in the engineering lab can learn the concepts in a practical way.
Читать дальше
NEW Basic Mechanics course
NEW Basic Mechanics course
Are you a teacher or do you work as an engineering researcher? EDIBON offers courses for teachers and #research staff. Take a look at the course Basic Mechanics and learn about #forces and vectors. #DiscoverEdibon #Engineering.
Читать дальше

ОБЩЕЕ ОПИСАНИЕ

This Simple Harmonic Motion Unit, "MSHU", allows many experiments using several pendulums and devices, such as different types of pendulums (simple, bifilar, trifilar and Kater’s pendulum) and springs.

Consists of a panel with a support that allows to hang different elements of study.

The study consists of letting freely swing different types of pendulums or a spring to check how different variables affect on their oscillation period. These variables to be studied are the mass hung from the pendulum or spring, and the oscillation length which can be easily modified with the tensors.

The Kater’s pendulum shows the relationship between simple harmonic motion and gravity, for prediction of gravity accurately.

The theory shows how to foretell the period of oscillation for a given pendulum or spring for comparison with actual results.

УПРАЖНЕНИЯ И ПРИМЕРЫ С ИНСТРУКЦИЯМИ

РУКОВОДСТВО ПО ПРАКТИЧЕСКИМ УПРАЖНЕНИЯМ ВКЛЮЧЕНО В РУКОВОДСТВО ПОЛЬЗОВАТЕЛЯ

  1. Simple harmonic motion of simple pendulum of different lengths and masses.
  2. Simple harmonic motion of bifilar pendulum of different lengths and masses.
  3. Simple harmonic motion of trifilar pendulum of different lengths and masses.
  4. Simple harmonic motion of a spring with different masses, and a simple spring rate test.
  5. Simple harmonic motion of the Kater’s pendulum.
  6. Calculation of gravity using the Kater’s pendulum.

БОЛЬШЕ ПРАКТИЧЕСКИХ УПРАЖНЕНИЙ ДЛЯ РАБОТЫ С УСТРОЙСТВОМ

  1. Theoretical determination of the period of a simple pendulum.
  2. Theoretical determination of the period of a bifilar pendulum.
  3. Theoretical determination of the period of a trifilar pendulum.
  4. Determination of the center of mass in pendulums with unbalanced masses.
  5. Determination of the mass center of a Kater’s pendulum with unbalanced masses.
  6. Theoretical determination of the period of the Kater’s pendulum, using the parallel axes theorem (Steiner’s Theorem).

КАЧЕСТВО

ПОСЛЕПРОДАЖНОЕ ОБСЛУЖИВАНИЕ

Запросить информацию